Deutsche Version
Homepage About me Satellitephotos Family Tree Sorbs Murphy's Laws Mandelbrot Mensa Riddles Links
Disclaimer
Where to retrieve good old Geometry
further riddles: Trivialities Analogies Integers Probabilities
- The height of the section of a drilling rig, which you can see with calm sea, amounts to 40 meters. With an hurricane it is torn from the anchorage. It falls over, and its outermost top sinks 84 meters far away
from its original point of view in the sea.
How deep is the sea here?
- If you draw two concentric semi-circles with a radius of 2 cm and 4 cm, cut out the surface between the two semi-circles and stick together the straight-line section.
Which volume does the truncated cone have, developed in such a way?
- Into a conical glass are filled successively mercury (density 13.59), water (density 1) and oil (density 0.91). The three liquids do not mix themselves and form three thick layers of equal thickness.
Does the glass then contain a larger mass water, oil or mercury?
- A windshield is cleaned by two windshield wipers with the length 50cm, which move around two points, whose distance amounts to 50 cm from each other. Thus everyone covers a semi-circle.
Which total area is wiped?
- It is given a triangle ABC, which is right-angled in B. M is a point on the hypotenuse, equivalent far from both sides of the triangle.
What is the value of: E =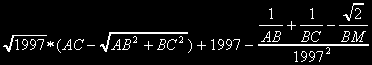
- Regard a N-cornered figure. There are how many diagonals, that are straight lines, which connect two not neighbouring corner points for N = 6,7,8?
- From the top of a prospect tower, which is in a height of 127.5 meters, you look to the horizon.
How far is it away, if you assume that the earth has a perimeter of 40000 km?
- Mr. and Mrs. Schulze go walking with their dog. Since everyone of them is eager to lead the line, they decide, to tie up the poor pet at two lines each of 1 m length.
If Mr. and Mrs. Schulze always walk with a distance of 1 meter to each other, which surface then the dog has at its free disposal?
- Assumed an orange (perimeter 30 cm) and the earth (perimeter 40000 m) would be accurate balls. Now you put one ring around the orange and one around the earth and then extend it by a meter.
When these rings have approximately the same distance from the surface all over, how does the distance then differ in case of the orange and in case of the earth?
- From my airplane window I can see a piece of an island, a part of a cloud and a little bit of the sea. The cloud covers the half window, thereby it hides a quarter of the island, whose visible remainder apparently fills out
still another quarter of the window.
Then, seen through the window, how much is the proportion of the sea covered by the cloud?
- In each corner of a small square room of 5 meters side length in a museum an armament is set up. The director means that they would come better into their own, if they were placed into a triangular niche (one in each corner), so that the in such a way changed room has 8 sides of equal length.
Which measure do these niches then have?
In case of questions or suggestions just write an Email to me.